The Skeleton
of Chaos
Tufts mathematician uses Ranger to uncover secrets of
dynamical systems
Story Highlights:
|
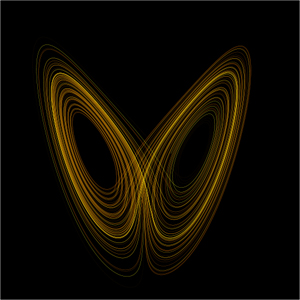
The butterfly wings of the Lorenz attractor have come to symbolize the shape of chaos. The form’s fractal structure corresponds to the long-term behavior of equations that describe convection rolls in the atmosphere, but that’s not why it’s famous. What’s startling about the shape is how it shows the state of a dynamical system evolving over time in a complex, non-repeating, but orderly pattern.
Many chaotic, non-linear dynamical systems have orbits of recurring activity — strange attractors that pull the system back when it gets too far off track.
Chaos, it seems, has an underlying structure.
Experts believe strange attractors like the Lorenz attractor tell us something about the mathematics of all dynamic systems, which include everything from pendulums to particle motions. In fact, scientists believe the secret to understanding turbulence — often called the last unsolved problem in classical mechanics — lies in these attractors, and something called the dynamical zeta function embedded in their forms
|
An icon of chaos
theory, the Lorenz attractor, |
In the early 1990s, Predrag Cvitanovic, professor of physics at the Georgia Institute of Technology, realized these problems could be solved through numerical simulation. He received the prestigious Alexander von Humboldt Prize in 2009 for his work in turbulence theory. But finding these orbits in real, complex systems has proved elusive because of the numerical difficulty of the equations.
With the emergence of petascale computing, researchers have begun moving toward the goal of unraveling the secret of dynamical systems.
Bruce Boghosian, professor and chair of mathematics at Tufts University, together with Peter Coveney and Luis Fazendeiro of University College London, uses the Ranger supercomputer at the Texas Advanced Computing Center (TACC), as well as other high-performance computing systems in the National Science Foundation’s TeraGrid to find unstable periodic orbits — recurrent hydrodynamic motions that scientists hope will eventually lead to a determination of the dynamical zeta function for turbulent systems.
“The work that we’re doing will give us a more fundamental understanding of the nature of turbulence in fluids, and in particular, a first principles understanding of how to take averages of observable quantities in a turbulent flow,” said Boghosian. “This is the most fundamental way to understand the problem of turbulence, and it’s only with machines like Ranger that we can do these kinds of simulations now.”
Turbulence is characterized by the co-existence of large scales of flow and very small eddies, with motion at every single length-scale in between. Direct numerical simulation of the Navier-Stokes equations (which describe the motion of a viscous fluid) is not possible for the high Reynolds numbers found in many engineering and industrial applications, so researchers use methods, such as large eddy simulation, wherein the smallest scales are not resolved. This is a very useful approximation that makes many calculations of turbulent phenomena possible, but it includes physical assumptions outside of those contained in the Navier-Stokes equations alone. It would be preferable to have an understanding of turbulence that was based entirely on the Navier-Stokes equations, according to Boghosian.
“The work that we’re doing will give us a more fundamental understanding of the nature of turbulence in fluids, and in particular, a first principles understanding of how to take averages of observable quantities in a turbulent flow...it’s only with machines like Ranger that we can do these kinds of simulations now.”Bruce
Boghosian, professor and chair of mathematics at Tufts University |
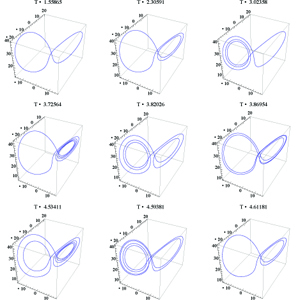
Unstable periodic orbits (UPOs) are based solely on these equations, and contain very useful information about the turbulent state. They are sometimes referred to as the “skeletons of chaos,” because they correspond to the underlying bones of dynamical systems.
The ability to computationally identify UPOs is based on an interesting discovery. In the real world, turbulent phenomena like ocean currents, wind racing over an airplane wing, or water passing through pipes, do not repeat cycles of motion. However, if the starting point and initial dynamics are just right, it’s possible for the motions to repeat and become regular.
“It’s ironic that these particular periodic orbits, which are flows that you’ll never see in the laboratory because they’re so unstable, are the most important things in determining turbulent averages of observables over flows that you will see in the laboratory,” said Boghosian.
In other words, by finding recurrent motions where the system is in a kind of balance, Boghosian derives information that describes the system in its natural chaotic state. To do so, he applies a novel technique to simulate the turbulent system represented by the Navier-Stokes equations, and to derive the initial conditions that lead to periodic motion. Starting off with an orbit that is periodic, but does not satisfy the Navier-Stokes equation, he “relaxes” the simulation to arrive at UPOs that represent the equations.
The method involves parallelizing the problem in both time and space, using HYPO4 software created by Boghosian’s research team, and storing all of the possible configurations in memory. This is a leap forward from simulations where only space is parallelized, while the simulation proceeds linearly.
“The petascale resources that are now available allow us to store the entire space-time configuration of the periodic orbit in memory,” explained Boghosian. “The common wisdom is that time is the one dimension that must be treated serially. This approach brings the benefits of parallelism to time as well as space.”
Using this framework, Boghosian and his colleagues have derived several UPOs, taking the first steps towards uncovering the dynamic zeta function for turbulent systems.
|
Evolution of gradient descent equations |
The project was performed across more than 33,000 processors on Ranger, displaying near-linear scaling. It used more than 18 million computing hours in 2008-2009, or the equivalent of 2,000 years on a single processor.
|
Bruce
Boghosian, professor and chair of |
The aims of the project are fundamental to science, but its conclusions have practical implications as well. Dynamical systems are omnipresent in our world, from the growth of forests to the action of neurons in the brain. All require a better, more fundamental understanding of the mathematics of chaos, which can be derived only by using high-performance computers.
In the future, Boghosian hopes to identify enough UPOs to determine the dynamical zeta function of turbulent systems and to create more efficient algorithms for finding these functions. He also has plans to curate a collection of unstable periodic orbits, and will create a portal allowing others to apply his methodology to similar problems.
In the meantime, Boghosian is operating his code at ever-larger scales. On the Intrepid machine at Argonne National Laboratory, and on Kraken at the National Institute for Computational Studies, the code exhibited linear scaling up to 65,000 cores, making it among the most powerful codes in computational science.
The natural world is governed by mathematics. For now, the order imbedded in nature’s complex mathematics is to be derived via parallel computers.
“Many of us want to see a truly reductionist understanding of turbulence and a method that is based solely on the Navier-Stokes equations,” said Boghosian. “This dynamical zeta function methodology holds the promise of giving us a fundamental understanding of these phenomena.”
Further reading:
1) L. Fazendeiro, B.M. Boghosian, P.V.
Coveney, J. Lätt, "Unstable Periodic Orbits in Hydrodynamics," Journal
of Computational Science, in press (2010).
2) P. Cvitanovic, R.
Artuso, P., Dahlqvist, R. Mainieri, G. Tanner, G. Vattay, N. Whelan, A.
Wirzba, "The Chaos Book".
This work
was supported by EPSRC grant EP/D051754/1 and the US NSF MRAC grants
TG-DMR070013N and TG-DMR070014N. Part of the work was supported by
AFOSR grant FA9550-04-1-0176 and ARO grant W911NF0410334; graphical
visualization was carried out on equipment purchased by NSF MRI grant
0619447. We are grateful to TACC for facilitating our early
friendly-user access to Ranger. We also acknowledge the support of the
ANL's Argonne Leadership Computing Facility for help and support in
accessing their resources, as part of a DOE INCITE allocation grant
supported by US Department of Energy under contract DE-AC02-06CH11357.
Aaron Dubrow
Texas Advanced Computing
Center
Science and Technology Writer
March 17, 2010
The Ranger supercomputer is funded through the National Science Foundation (NSF) Office of Cyberinfrastructure “Path to Petascale” program. The system is a collaboration among the Texas Advanced Computing Center (TACC), The University of Texas at Austin’s Institute for Computational Engineering and Science (ICES), Sun Microsystems, Advanced Micro Devices, Arizona State University, and Cornell University. The Ranger and Lonestar supercomputers, and the Spur HPC visualization resource, are key systems of the NSF TeraGrid (www teragrid.org), a nationwide network of academic HPC centers, sponsored by the NSF Office of Cyberinfrastructure, which provides scientists and researchers access to large-scale computing, networking, data-analysis and visualization resources and expertise.